Mirror and light experiment
Wednesday, July 19, 2006
I started using eye glasses 3 years ago. I don't like wearing them, but I have to since I'm required by law to wear them while driving--this is the only time I use them.
My prescription is for nearsightedness, which is an eye defect that focuses images incorrectly in front of the retina (I call it premature focusing), thus making far away images to appear fuzzy.
A few hundred years ago, someone figured out that using a diverging lens would force the light coming into the eye to properly diverge before hitting the cornea thus focusing properly in the retina--the cornea is just a natural "lens" inside our eyes.
Because of my defective eyes, I started thinking about plane or flat mirrors and how images reflect "off" of them.
I devised a simple experiment that goes like this:
- With my glasses on, I focus on an object far away--say a tree located about 500 meters away.
No surprises here, I can see the tree clearly.
- I take my glasses off and, appropriately, I can still see the tree, however, it looks fuzzy because of my nearsightedness.
At this stage I did a little thought experiment to answer the question "what would I see if I turn 180° and place a mirror in front of me, say 40 cm, so that I can see the tree behind me?" (Remember, I can clearly see things that are close to me.)
I started thinking about optics and the reflection of light and I immediately concluded that I would see the same tree as perfectly as I would with my glasses on. I hypothesized that the reflection of the tree is "created" 40 cm in front of me, therefore no fuzziness. I imagined the light bouncing off the mirror to hit my eye and only traveling 40 cm--I had invented another cure for nearsightedness, I thought.
At this point, I grabbed a mirror to corroborate the results of my thought experiment.
Was I right or was I wrong?
Some of you may already know that my hypothesis is wrong. It sounds logical (to me at least), however, human logic is not the same as nature's logic. I couldn't figure out why I was wrong.
(An interesting side note: if you don't suffer from nearsightedness, you can't confirm my results and you will have to take my word for it.)
The question to answer now is why is this so, i.e., why is the reflected image in the mirror, which is 40 cm in front of me, fuzzy without my glasses but not fuzzy if I put them on?
While it is true that the mirror is in front of me at a distance of 40 cm, the actual image reflected is still 500 m in front of me.
To prove the fact that the image in the mirror is actually 500 m away in front of me, I had to remember how mirrors actually work.
I eventually had to open up my undergrad Physics book to start my quest to understand why the empirical results differed from my thought results.
I have to say that It was quite a discovery for me, as I had never noticed this phenomena since eye glasses were not part of my every day life until 3 years ago.
I'll spare you all the theory of optics and quote directly from my book what is actually happening to the reflected image. Images formed by flat mirrors have the following properties:
- The image is as far behind the mirror as the object is in front.
- The image is unmagnified, virtual, and erect. (By erect we mean that, if the object arrow points upward, so does the image arrow.)
- The image has right-left reversal.
Note that these are not laws, but properties.How do these properties help me in answering my question?
Note property number 1: what it is saying is that any reflected image in front of a mirror generates a virtual image "behind" the mirror, and the distance of this virtual image is exactly the same distance as the real image in front of the mirror.
I think a diagram is helpful here--note that this diagram assumes the correct "particle" theory of light propagation so that "rays" of light propagate in straight lines (more on this below):
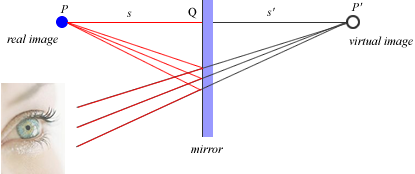
Obviously, there are more than three "rays" of light bounced off from the mirror as generated by the real image, however, simplicity is key to understand what is going on. What you should note, though, is that the distance
s is the same as
s', or the distance relative to the mirror for the real image is the same as the virtual image (which is the reflection of the real image).
There is one rule we can borrow from Geometry to support the statement "the real image's distance relative to the mirror is the same as the virtual image's distance relative the mirror": we can confidently say that because the triangle PQR is
congruent to the triangle P'QR, then
s = s' and
h = h'. See the image below to see what I mean by congruent:
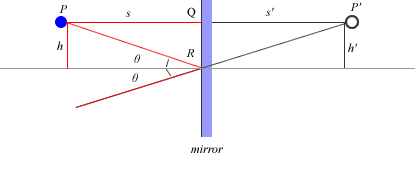
So if you believe in all that Geometry sorcery, you will agree with me that such statement makes sense, and you will also agree that the virtual image is actually located at s' distance behind the mirror, thus making total sense why without my glasses the image appears fuzzy.
If you are still reading carefully, you will object to this explanation and still ask why is this so?
Alright, let me continue.
I will ask us to imagine "rays" of light being bounced off the virtual image and that these rays do travel in a straight line.
Using Geometry again, I can confidently say that the angle of reflection of the real image coincides with that of the virtual image. Therefore the angle between the ray and its reflection is the same, namely θ or "theta."
Hence, by tracing lines around the diagram we can calculate what this angle should be, because we know where in the mirror the virtual image resides and we also know the real image's location. (Because of this geometry we can do other interesting things with reflected images--out of scope right now, but worth mentioning.)
So, Physicists will explain that the bounced "rays" of light are spreading as they should, therefore hitting your eye as if the image was located "behind" the mirror--in other words, what you are actually looking at is the virtual image after all, and not the "flat" reflection in the mirror, i.e., light is not actually "drawing" the reflection on the mirror, although it looks like it is because of the flatness of the mirror.
Now, I think, it should be clear why my thought experiment's result was wrong: the tree was not really 40 cm in front of me, but 500 m in front me "behind" the mirror. Silly me.
Am I happy with my own explanation? Actually, no.
After all the research and silly geometry tricks, I am not satisfied as to why this happens the way it happens. I think it is logically explained, however, why is property number 1 above true?
The problem with trying to understand the true nature of things leads to
really trying to understand, which is in itself frustrating because nature is not that simple. It is similar to the easy questions to ask that have no real satisfactory answer, for example, "why are we here?"
Anyway, looking further into why property number 1 above holds true has led me into more obscure and weird explanations of nature: Quantum Theory.
This brings in a bit of problem for me because Quantum Theory is not that simple to understand, and I probably need some extra mathematical training, beyond my Mathematics degree, to do real calculations that will satisfy my curiosity.
However, I found Richard Feynman's book
QED. The Strange Theory of Light and Matter very useful. Though, after reading it, I still don't have a satisfactory answer to my original question.
So nature is weird, but it is simple if you believe what theoretical physicists think is true about QED: according to Feynman, everything around us can be explained with the following three actions between photons and electrons (Chapter 3, page 85):
- A photon goes from place to place.
- An electron goes from place to place.
- An electron emits or absorbs a proton.
It is quite remarkable and seemingly possible that these three "simple" actions explain everything (except for gravitation) about our world, from chemistry to computers and even images reflecting off of mirrors.
So there is hope for me to understand why I was wrong and how my deficiency led me to waste my time wondering and creating thought experiments.
Can anyone clearly explain and fully convince me of why far-away reflected images from a mirror are still fuzzy to non-eye-glasses-wearer nearsighted people? I would really, really like to know...
(Diagrams are based on Chapter 35 and 36 of
Physics for Scientist and Engineers with Modern Physics, Third Edition, by Raymond A. Serway.)
Comments: